For a given FREQUENCY, the critical band is the smallest BAND of frequencies around it which activate the same part of the BASILAR MEMBRANE. Whereas the DIFFERENTIAL THRESHOLD is the just noticeable difference (jnd) of a single frequency, the critical bandwidth represents the ear's resolving power for simultaneous tones or partials.
In a COMPLEX TONE, the critical bandwidth corresponds to the smallest frequency difference between two PARTIALs such that each can still be heard separately. It may also be measured by taking a SINE TONE barely MASKed by a band of WHITE NOISE around it; when the noise band is narrowed until the point where the sine tone becomes audible, its width at that point is the critical bandwidth. See: RESIDUE.
In terms of length (see diagram under BASILAR MEMBRANE) the critical bandwidth is nearly constant at 1.2 mm, within which are located about 1300 receptor cells, and is generally independent of intensity (unlike COMBINATION TONES). Twenty-four critical bands of about one-third octave each comprise the audible spectrum (see Appendix E).
Simultaneous tones lying within a critical bandwidth do not give any increase in perceived LOUDNESS over that of the single tone, provided the sound pressure level remains constant. For tones lying more than a critical bandwidth apart, their combination results in increased loudness (see Appendix F).
When two tones are close together in frequency, BEATS occur, and the resulting tone is a fusion of the two frequencies. As the frequency difference increases, roughness in the tones appears, indicating that both frequencies are activating the same part of the basilar membrane. Further apart, the two frequencies can be discriminated separately, as shown below by DfD, whereas roughness only disappears at a frequency separation equal to the critical bandwidth DfCB. At this point, the two frequencies activate different sections of the basilar membrane. This phenomenon only applies to monaural listening with pure tones. With DICHOTIC listening, the basilar membrane of each ear is activated separately, and therefore no roughness results. With complex tones, frequency discrimination is improved but the critical bandwidth remains the same for each of the component partials.
Compare: Constant Q FILTER, FOURIER ANALYSIS, LAW OF SUPERPOSITION, MEL, SOUND ANALYSER.
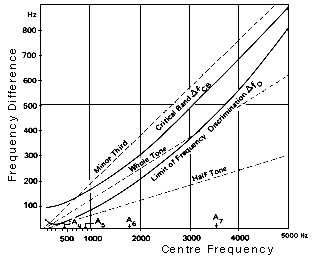
Critical bandwidth DfCB (after Zwicker, Flottorp and Stevens) and limit of frequency discrimination DfD (after Plomp) as a function of the centre frequency of a two-tone stimulus. Various octaves of the pitch A and the frequency difference corresponding to three musical intervals are shown for comparison (from Roederer, Introduction to the Physics and Psychophysics of Music, Springer, 1975, p. 30, used by permission).
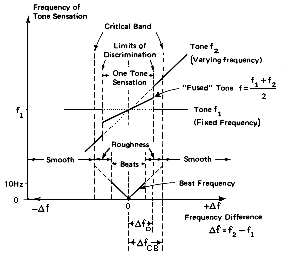
Schematic representation of the frequency (shown in heavy lines) corresponding to the tone sensations evoked by the superposition of two pure tones of nearby frequencies (from Roederer, Introduction to the Physics and Psychophysics of Music, Springer, 1975, p. 29, used by permission).
![]() Sound
Example: Two sine waves, one fixed at
400 Hz, the other ascending from 400 Hz to 510 Hz at which point
it is separated from the first by a critical bandwidth.
Sound
Example: Two sine waves, one fixed at
400 Hz, the other ascending from 400 Hz to 510 Hz at which point
it is separated from the first by a critical bandwidth.
home