The FREQUENCY content of a sound or audio SIGNAL, often displayed as a graphic representation of amplitude (or INTENSITY LEVEL) against frequency. Three-dimensional displays of a spectrum add the time variation on the third axis (see below). The spectrum of a sound is a primary determinant of its perceived TIMBRE.
A PARTIAL spectrum consists of discrete frequencies known as OVERTONEs, HARMONICs or INHARMONICs. A continuous spectrum consists of NOISE components. The spectrum of a sound may be determined by a SOUND ANALYSER or by FOURIER ANALYSIS and is distributed over the audible range (20 to 20,000 Hz). A partial spectrum is also known as a line spectrum, where discrete frequencies are present. A continuous spectrum, on the other hand, shows frequencies continuously distributed over the audible range.
See: diagrams under BROAD BAND NOISE, FOURIER ANALYSIS, INFRASONIC, SPECTROGRAPH, WHITE NOISE.
Analyzing the spectrum of a sound is a way of understanding its behaviour in the frequency domain, as opposed to its behaviour in the time domain, according to its WAVEFORM or ENVELOPE. The auditory system is designed to balance the simultaneous resolution of detail in both domains, as expressed by the LAW OF UNCERTAINTY.
The spectral envelope refers to the contour or shape of the spectrum, particularly when it shows the maximum strength of each frequency component during the sound.
The spectrum of a sound may be altered electronically by FILTERing or EQUALIZATION.
Spectrum may also refer to a RANGE of frequencies, as in the audio spectrum (see AUDIO FREQUENCY) or the RADIO SPECTRUM.
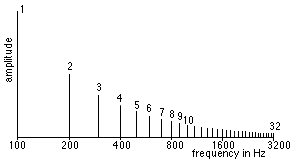
The first 32 partials of a HARMONIC SERIES shown as a line spectrum. The amplitude of each partial is inversely proportional to the partial number.

Line spectrum of the partials of a viola string, omitting their time variation.
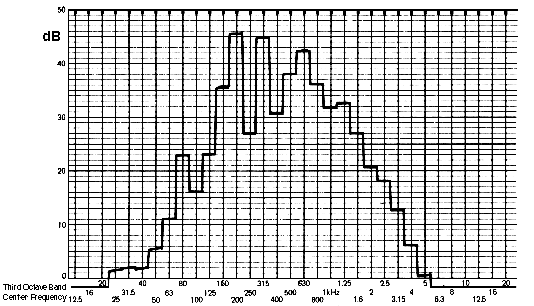
Third-octave spectrum of the Salvatore Mundi bell, Salzburg. Peak intensities occur in the frequency bands centred on 200, 315 and 630 Hz, with a fundamental about 80 Hz.
Sound Example: Salvatore Mundi.
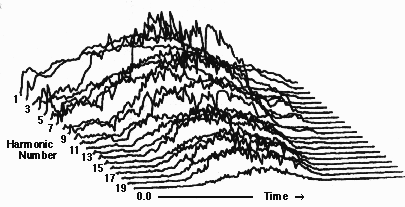
Three-dimensional plot of a trumpet tone showing the amplitude envelope of the first 20 harmonics. From J. Beauchamp & A. Horner, "Synthesis of trumpet tones using a fixed wavetable and a centroid-controlled second order filter," Proceedings of the 1994 International Computer Music Conference, used by permission of the authors.
home