The representation of a PERIODIC sound or WAVEFORM as a sum of Fourier components (i.e. pure SINUSOIDAL WAVEs). According to the FOURIER THEOREM, periodic sound may be shown to consist of SINE WAVEs in the HARMONIC SERIES, where the Fourier coefficients give the AMPLITUDE and PHASE angle of each component.
Fourier analysis may be performed mathematically if the expression f(t) describing the waveform or COMPLEX TONE is known, or else by converting the sound to digital form by a computer which then analyzes it. The average SPECTRUM of an instrument may be obtained in this way by analyzing it during a representative section of its STATIONARY STATE. However, if every period of the sound is analyzed, it will be seen that the spectrum is always changing in time, i.e. the harmonic components in the spectrum are constantly changing in amplitude. A more general form of analysis for transferring a time-domain signal to the frequency domain is called the Fourier transform.
Compare: FOURIER SYNTHESIS, HELMHOLTZ RESONATOR, LAW OF SUPERPOSITION, SIMPLE HARMONIC MOTION, SOUND ANALYSER, TIMBRE.
As well, it appears that the ear performs Fourier analysis on incoming sounds, in that separate harmonics may be distinguished up to the point where they tend to fuse together, that is, at the point where the harmonics are separated by a distance equal to the CRITICAL BANDWIDTH, indicating that they activate the BASILAR MEMBRANE in the same region. However, in most cases only the first 5 to 7 harmonics may be heard separately, and only in sustained tones. See: RESIDUE.
The diagram below shows the results of Fourier analysis of every period of a short trumpet tone (0.16 sec. at 550 Hz). The time behaviour of the first seven harmonics is shown from right to left as the sound progresses. At no time is the spectrum perfectly stationary. The greatest variation is during the onset TRANSIENTs shown at the right.
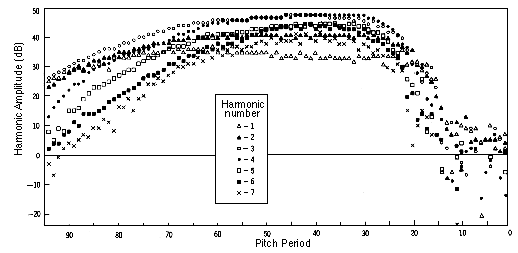
Pitch synchronous analysis of a trumpet tone. These measurements are for a short tone (0.16 sec.) with a fundamental frequency near 550 Hz (C sharp). Time, measured in units of one pitch period, runs from right to left because the pitch-synchronous programs give more information on the attack (on right of plot) when proceeding backward in time. Seven harmonics are shown here (from M. Mathews and J.C. Risset, "Analysis of Musical-Instrument Tones", Physics Today, 1969, vol. 22, no. 2, p. 26, used by permission of the authors and the American Institute of Physics).
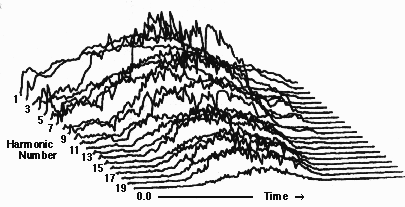
Three-dimensional plot of a trumpet tone showing the amplitude envelope of the first 20 harmonics. From J. Beauchamp & A. Horner, "Synthesis of trumpet tones using a fixed wavetable and a centroid-controlled second order filter," Proceedings of the 1994 International Computer Music Conference, used by permission of the authors.
home