Math 178 Homework and Assignments
Short writing assignment #1. Draft due Friday (in
class), May 18, final version due Friday May 25
Quantitative assignment #1. Due: Friday June
1
- (1) Recall the decomposition of the
triangle into 4 smaller triangles of size 1/2 the whole triangle (as when
we discussed the Sierpinski triangle). Number the small triangles 1 (bottom
left), 2 (bottom right), 3 (top), 4 (centre).
- (a) Make a sketch of the triangle and indicate on the sketch
address regions 24, 144, 1234.
- (b) Use the template below
to make a sketch of the image of the triangle after removing all address
regions UP TO ADDRESS LENGTH 5 that contain a '21'.
Please use this template for your sketch (it will be easier for us to
mark). Indicate on your sketch the addresses of these
regions. It will be easier to shade the regions missing rather
than the regions remaining.
(Start with locating all address regions of
length 2 that contain '21', then all address regions of length 3 that
contain a '21', for example 121, and 211, then all address regions of length
4, etc.). Is this object self-similar? Why or why not?
Here's an image of
the triangle decomposed into smaller triangles; you can use this to make
sketches for your answers.
- (2) Use the formula for the sum of a geometric series;
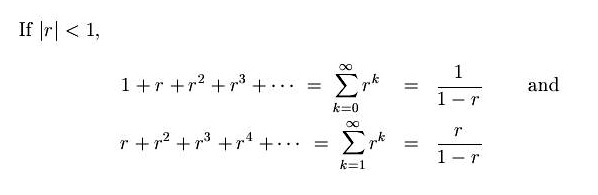
to show that all the area of the
original triangle is removed during the construction of the Sierpinski
triangle.
- (3) Recall the construction of the Cantor (middle thirds) set; Construction of the
Cantor set, and the description of it in terms of ternary expansions of
numbers (i.e., the Cantor set is the numbers in [0,1] whose ternary expansion
contains no '1'; Cantor
set and ternary expansions.
Also, recall the method to calculate
the ternary expansions of numbers;
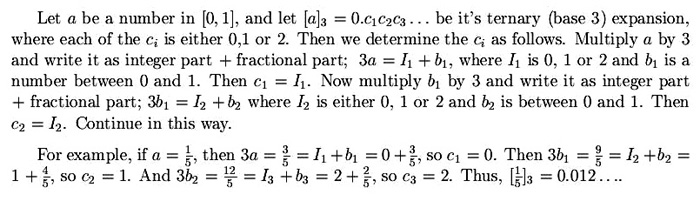
- (3a) Determine whether the following numbers are in the Cantor
set or not; 5/7, 37/40, Pi/4 (for this last one, just follow the same
procedure as above).
- (3b) Give a description of how to obtain the Cantor "middle
fifths" set by first decomposing [0,1] into 5 pieces (with end points at 0,
1/5, 2/5, 3/5, 4/5, 1), and removing the middle fifth segment (2/5, 3/5).
Then decomposing the remaining 4 pieces each into 5 sections and removing
the middle fifths from each of these, etc.
- What is the length of all the intervals removed?
- Describe the Cantor middle fifths set in terms of expansions of
numbers in base 5.
- Are the following numbers in the Cantor middle fifths set or not; 1/3,
(square root of 2)/5.
- (4) Suppose the graph of log(u) vs log(1/s) for a
curve is a straight line with slope 3/2. If u(1)=100, what is the length of
this curve at the scale 1/4? What is the length of this curve?
- (5a) Can a curve of finite length have length exponent
d>0? Explain.
(5b) Can a curve of infinite length have
length exponent d=0? Explain.
- (6) Recall the Square von Koch
curve. It was constructed by repeating this
procedure of adding squares.
Calculate the length exponent d for this
curve.
- (7) Recall that the covering dimension, or fractal dimension, of a
set is denoted by D. Compute the covering dimension D of the following
sets.
- The Cantor middle fifths set (see problem (3b) ). Is this set more or
less complicated that the Cantor (middle thirds) set?
- The Square
von Koch curve. Compare this with your answer for the length exponent d
you found in question (6).
- The fractal obtained by following this template
(variation on Sierpinski; keep only the middle 4 triangles of the 16
triangle decomposition of the full triangle). Is this object more or less
complicated that the Sierpinski triangle fractal?
- This square
variation fractal, which was obtained from this template.
Writing assignment #2. Draft due Friday (in class),
June 15, final version due Friday June 22
Write a 2 - 3 page (single-spaced) review of the video "Fractals: An
Animated Discussion" that we watched in class friday (June 8). This is meant
to be a review to people who are interested in learning about fractals and
chaos (general public). Your review should discuss what the video covers as
well as your opinions as to it's effectiveness. For your reference, here's a summary of the
topics covered. Note also that the texbook discusses the material covered
in the video.
The video is on reserve in the library (under
instructor's name and class number); you can borrow it for 2 hours and watch
it there.
Please hand in your draft (outline) in friday's class, June
15. I will return them next wednesday and the final version will be due
Friday, June 22.
Quantitative assignment #2.
Problems 1 - 6 due Friday, June 29 in class, Problems 7,8 due Wednesday July 4. Solutions will
be provided at that time no late assignments will be accepted.
- (1) Recall our definition of fractal numbers; A number
D is a fractal number if there is a set whose fractal
dimension is D.
- (1a) What is the smallest and largest fractal number that one may
encounter?
- (1b) If a set S is drawn on a blackboard, explain why
it's fractal dimension must be between 0 and 2, including perhaps 0
and 2.
- (1c) Construct sets with the following fractal dimensions;
log(5)/log(9), 1.5, log(17)/log(4).
- (1d) Use consistent coverings to construct sets which
we haven't seen before in class, with the following properties;
- A set whose fractal dimension is 1 but is entirely dust (i.e.,
contains no lines).
- A set with fractal dimension log(5)/log(4) but is not
self-similar.
- (1e) Explain in as much detail as you can, what are the possible
fractal numbers. (Can any number be a fractal number? Can you give
a formula for a possible fractal number? Remember, if you say that
D is a fractal number you must find a set S whose fractal
dimension is D, which means you have to find a covering for
S which gives dimension D.)
- (2) Recall that an IFS W is made up of affine
transformations wi = Ai + vi where
Ai is a 2 by 2 matrix and vi is a (2
dimensional) vector (the 'shift vector').
- Write out the matrix A and shift vectors v for the
following transformations (refer to my notes
on affine transformations);
- Rotation 20o clockwise followed by contraction in the
x-direction by 0.6 and contraction in the y-direction by 0.9 (no
shift).
- Dilation by 0.4 followed by reflection across the x-axis and then
shifted so that the top right corner of the image of the square lies at
the location (1,1).
Find the fixed points of these
transformations (verify that these points p are indeed fixed points
by computing w(p) and seeing that this is equal to
p.)
Added: Sketch the image of the
square under these transformations, and also compute the image of the square
by calculating the locations of the vertices (0,0), (1,0), (1,1),
(0,1) under these transformations.
- (3) Let W be the IFS for the Cantor Maze (this can be
found in section 5.2). Make a sketch of the first 3 iterations of this IFS
when the initial image S is;
- the diagonal line from the bottom left to upper right corner of the
initial square
- a circle centered at the midpoint of the initial square and diameter
equal to the diameter of the square (so the circle just fills the initial
square)
- an equilateral triangle that fills the initial square (like the outlins
of Sierpinski's triangle)
Which one of these initial images do
you think would produce the image of the fractal the fastest when the IFS is
iterated?
- (4) Use the Visual Basic program Fractal Pattern or the
Chaos Game Applet that can be found on the software page to create
unique (i.e., ones we haven't seen before) fractals with the following
properties. Please hand in an image of the fractal along with the parameters
of the IFS you used to create them. (Here are
instructions for using and producing images of fractals with these
programs.)
Here are
some notes on affine transformations (handed out in class).
Go here for examples of
iterations of IFS created with the Fractal Pattern Visual
Basic Program (which you can download), and here for examples
of how to create certain affine transformations using this program.
*******************************************************************************
In case you can't get the Visual Basic program Fractal Pattern running (eg., your OS is Mac), then you can use
the Fractal Movie applet to view the blue print of the IFS and the fractal. Here you just enter the IFS you
are interested in as the start pattern and as the end pattern (see the instructions posted with
the applet). This way the IFS doesn't change while the program
is running. To get an image of the fractal, follow the instructions
in here regarding printing the image
using the chaos game applet.
********************************************************************************
- Fractals 1,2,3 Reproduce the fractals in Figure 5.28 of the text
(Section 5.3). That is, figure out what the IFS is for each and then run the
programs to draw.
- Fractal 4 A cloud-like fractal. Try to make one that looks like
this cloud. (Hint:
start with the spiral fractal (swirls) and add more 'lenses' to this IFS.)
- Fractal 5 A new, interesting, fractal! (More marks will be given the more
complicated the fractal is, i.e., number of transformations and if they are more than
just dilations. Also, I will display these in class later on.)
- (5) Consider these two fractals; Mess1 and
Mess2. Here is a
blue print (image of the square under one iteration of
the IFS). Which one of these fractals is the fixed point of this IFS? Justify your answer.
(You may also want to consider this second iterate of the IFS.)
- (6) Consider the IFS for the Crystal 4 fractal;
W = w1 U w2 U w3 U w4 (see Figure 5.14 in
Section 5.2, or look it up with the applets). The contraction rates for each transformation
are; w1, w2, w3 ; 0.255, w4 ; 0.74.
(If the matrix entries are a,b,c,d like we have been denoting, then the rate of contraction
for the matrix is square root of ad-bc).
Assume that your computer can draw 10,000 squares per second, and the size of the initial square is 1000 by 1000
pixels. Calculate how long it will take your computer to draw this fractal by iteration of the IFS.
Can your computer draw this fractal this way?
- (7) Consider the Sierpinski Triangle IFS with probabilities p1 = p2 = 0.2,
p3=0.6.
(7a) If 10,000 game points are used in the Chaos Game, determine how many
game points you would expect to find in each of the subtriangles with address length 3.
(7b) Estimate how long it would take this Chaos Game to draw the fractal (i.e., how long of a game
sequence is needed). Run the Chaos Game applet with these probabilities and comment on how
many game points are needed there and compare to your answer. (To answer this question, use the data
given in the table below about the sizes of the canvases in the chaos game applet.)
The sizes (in pixels) of the canvases that contain the
image produced by the chaos game applet are;
| Version of applet
| Canvas size (pixels)
|
| 640 X 480 (VGA)
| 304 X 304
|
| 800 X 600 (SVGA)
| 380 X 380
|
| 1024 X 768 (XVGA)
| 500 X 500
|
| 1280 X 1024 (UVGA)
| 645 X 645
|
1400 X 1050
| 800 X 800
|
- (8) Consider the Full Triangle IFS for this question (that is, the same IFS as for
Sierpinski but add in the middle, 4th (upside down), triangle).
(8a) Explain why when the Chaos Game is played with the sequence 4,4,4,. . . . (i.e., 4 repeating) that the game points
eventually converge to the fixed point of w4. Verify the location of this point by solving
the equation w4(p)=p for the fixed point p (you can look up the parameters for this
IFS by checking one of the applets) ;
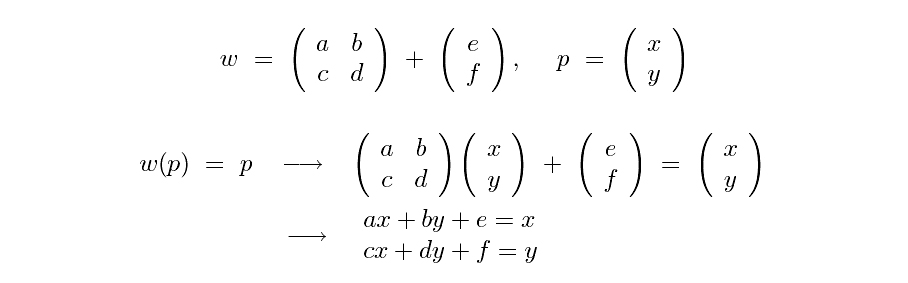 .
.
(8b) Explain that when the Chaos Game is played with the sequence 1,2,1,2,1,2,. . . (i.e., 1,2 repeating) that two
points result (eventually). Sketch the location of these points on the triangle, then determine the
exact location by considering the fixed points of w12 = w1w2
and w21 = w2 w1 (these are compositions). That is, solve the equations
w1(w2(p)) = p, etc.
(8c) What image results if the Chaos Game is played with the
sequence 1,2,3,4,1,2,3,4, . . . (i.e., 1,2,3,4 repeating)? Sketch the locations of the game points.
How would one determine the
exact location of these game points? (just explain, you don't have to actually find these points)
End of assignment #2
Writing assignment #3. Due Wednesday, June 27.
Midterm test; Friday July 6 (2 hours)
The midterm test will cover all the material we have discusses in the course so far (all
about fractals). You can expect quantitative questions similar (but not necessarily identical)
to the quantitative assignment problems. In addition, there will be 2 or 3 short written
questions (one or two paragraphs each) concerning the theory discussed in the course.
Please bring a calculator and ruler.
Writing assignment #4. Due: July 25.
Group presentations about sections of articles on chaos that were handed out in class. See
here for details about this assignment and the evaluation rubric (this has been
updated from what was handed out in class wednesday).
Groups will work on this at next weeks' seminar (July 18). Presentation will be due at the seminar on July 25.
I passed around two books that give `popular' accounts of the development of chaos theory, and which may be useful
references here;
Chaos: Making a New Science, by James Gleick
Does God Play Dice?, by Ian Stewart.
Quantitative assignment #3.
Due: Friday, August 3 in class (no late assignments; solutions will be provided then)
(1) Here's plots of the function ga(x)=x3+ax for a = -2, 0, 2;
plots of ga(x)=x3+ax (the diagonal line y = x is
also plotted).
(1a) Use graphical iteration to determine the stability of each of the fixed points. Also, determine
the stable sets Ws(p) of each fixed point.
(1b) Construct both the bifurcation diagram and the final state diagram for ga(x) for
-2 < a < 2.
(2) Sketch the graph of a function
that has the period 4 orbit {1,-2,3,0} (in that order!).
Verify by graphical iteration that this function does indeed have
that period 4 orbit. (Hint: you want to first plot the points 1, -2, 3, 0
along the x-axis and then place the graph above these points at the appropriate location.)
(3) Using the Logistic Movie applet,
find a stable (prime) period 6
orbit for the logistic equation near parameter valus a=3.6 (record the exact value of
a you used). Include a sketch or screen shot of the histogram of the orbit at this value.
Then use the Bifurcation applet to locate (precisely) all points in this stable period 6 orbit
(click on with mouse) and all points in the unstable period 6 orbit. Verify, by iterating
the logistic equation with your calculator, that these points do form period 6 orbits
(include the results from your calculator). Run the
Graphical Iteration applet with this a value and with a point in the stable period 6 orbit.
Make a sketch or take a screen shot of the 'staircase' path of this period 6 orbit
(graphical iteration of the orbit). Label the points in the orbit along the x-axis.
(4) Suppose ga(x) is a family of functions whose orbits
(for various values of the parameter a) go through a sequence of period-doubling bifurcations
like we observed for the logistic family of functions. Let a1 be the parameter value
where the fixed point (period 1 orbit) bifurcates into a period 2 orbit, a2 the
parameter value
where the period 2 orbit bifurcates into a period 4 orbit, etc.
"Universality" says that period-doubling takes place at a common rate, namely that the ratios of the distances between
successive bifurcations is nearly constant at the value d for a wide variety of dynamical systems;
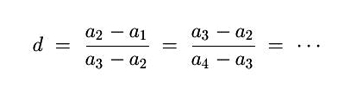 .
.
Here, d is the Feigenbaum constant; d = 4.6692.... We say that the system has reached chaos
when it reaches the "last" bifurcation point ainfinity, also
referred to as the Feigenbaum point (at which the periodic orbit now has
infinite period). Beyond this value of a orbits have aperiodic, or ergodic, behaviour.
(This is mentioned by David Ruelle in his article on turbulence that I handed out for group presentations. See also
Sections 11.2 and 11.3 in the text.)
- (4a) Verify that the period-doubling rates for the logistic equation nearly satisfy this
universality relation (you can look up the 'exact' values of the bifurcations in the text in Section 11.2).
- (4b) If we know the values of a1, a2 (or any 2 consecutive
bifurcation points), we can estimate the parameter value ainfinity where the onset of
chaos occurs in the following way;
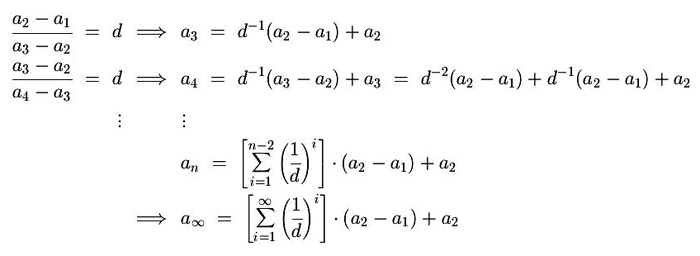 .
.
Use this formula to estimate the Feigenbaum point for the logistic equation and compare this value to what
you measure on the final state diagram.
- (4c) A dynamical system changes as the surrounding temperature T changes. Initially, you observe a
steady state of the system. As the temperature increases you observe that the system switches to oscillating
between two states at
T = 70C.
Then at T= 100C you observe that the system begins to oscillate between 4 different states. As the
temperature continues
to increase the system goes through a period-doubling sequence of bifurcations into more and more
complex oscillations. Assuming Universality, at what temperature would you expect
to observe the onset of chaos in this system?
(5) Show that the saw tooth transformation has an ergodic orbit.
(6) Use symbolic dynamics to find all period 2 points of the logistic equation f4(x).
(First find the symbol sequences (binary expansions), then transform these to numbers (hint: they are simple
fractions),
then transform these numbers via the function h(x) into numbers for the logistic equation.)
Verify
the answers by iterating the logistic function.
Last updated: July 26
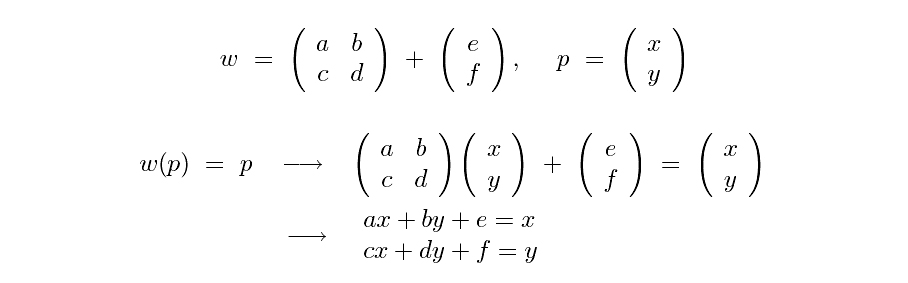 .
.