Representing the cube as a subgroup of $S_{48}$
Label the facets of the Rubik's Cube as shown in the diagram.
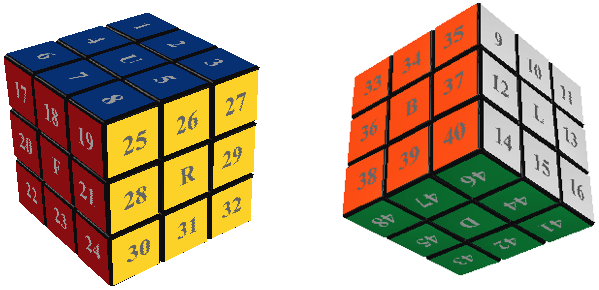
It may help to view this labeling on the flattened cube as follows.
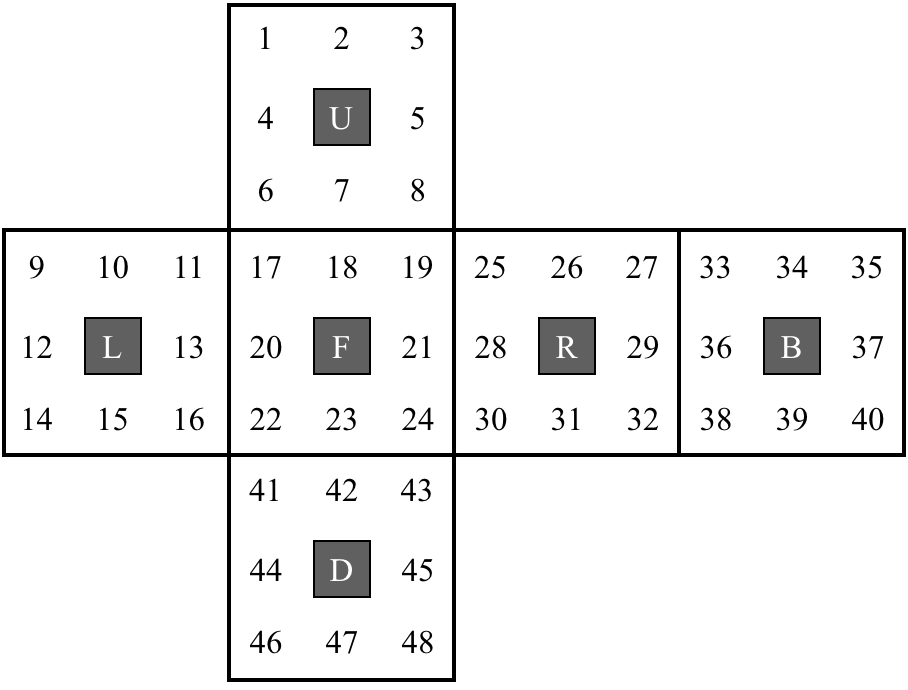
The permutations corresponding to each of the basic moves of the Rubik's Cube are: $$\begin{array}{l} R = (25,27,32,30)(26,29,31,28)(3,38,43,19)(5,36,45,21)(8,33,48,24) \\ L = (9,11,16,14)(10,13,15,12)(1,17,41,40)(4,20,44,37)(6,22,46,35)\\ U = (1,3,8,6)(2,5,7,4)(9,33,25,17)(10,34,26,18)(11,35,27,19)\\ D = (41,43,48,46)(42,45,47,44)(14,22,30,38)(15,23,31,39)(16,24,32,40) \\ F = (17,19,24,22)(18,21,23,20)(6,25,43,16)(7,28,42,13)(8,30,41,11)\\ B = (33,35,40,38)(34,37,39,36)(3,9,46,32)(2,12,47,29)(1,14,48,27) \end{array}$$ $R^{-1}, L^{-1}, U^{-1}, D^{-1}, F^{-1}, B^{-1}$ correspond to the inverses of these permutations.
Since the centre's of the cube are fixed by these moves then any two of these moves are inequivalent. This means that $RC_3$ can be represented by the subgroup of the Symmetric group $S_{48}$ generated by these permutations: $$RC_3 = \langle \mathrm{R}, \mathrm{L}, \mathrm{U}, \mathrm{D}, \mathrm{F}, \mathrm{B} \rangle.$$ We can define $RC_3$ in SageMath as follows.